Embark on a journey into the realm of absolute value equations and inequalities quiz part 2. This comprehensive exploration delves into the intricacies of absolute values, equipping you with the knowledge and techniques to conquer these mathematical challenges. Prepare to unravel the mysteries of absolute values, unraveling their properties and applications in a captivating and thought-provoking manner.
This quiz will test your understanding of absolute value equations and inequalities. You will be asked to solve equations and inequalities, and to apply your knowledge to real-world problems. By the end of the quiz, you will have a strong foundation in absolute value equations and inequalities.
Absolute Value Equations
Absolute value equations are equations that involve the absolute value of an expression. The absolute value of a number is its distance from zero on the number line. For example, the absolute value of 5 is 5, and the absolute value of -5 is also 5. Absolute value equations can be solved using the following properties:
- The absolute value of a number is always non-negative.
- The absolute value of a negative number is the same as the absolute value of its positive counterpart.
- If |x| = a, then x = a or x = -a.
To solve an absolute value equation, we first isolate the absolute value expression on one side of the equation. Then, we use the properties above to solve for x.
Absolute Value Inequalities
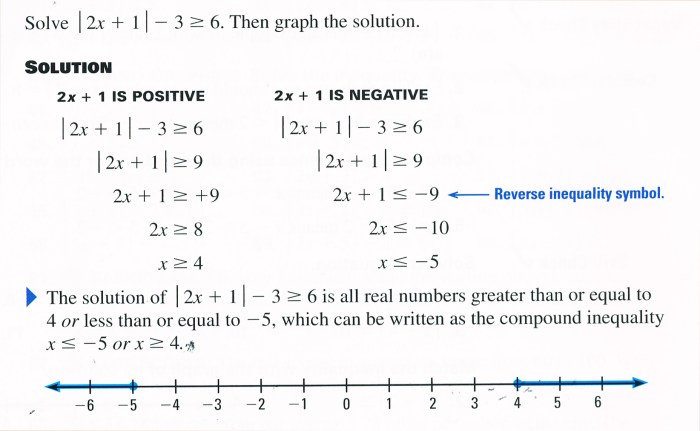
Absolute value inequalities are inequalities that involve the absolute value of an expression. Absolute value inequalities can be solved using the following properties:
- The absolute value of a number is always non-negative.
- The absolute value of a negative number is the same as the absolute value of its positive counterpart.
- If |x| < a, then -a < x < a.
- If |x| > a, then x < -a or x > a.
To solve an absolute value inequality, we first isolate the absolute value expression on one side of the inequality. Then, we use the properties above to solve for x.
Solving Absolute Value Equations and Inequalities: Absolute Value Equations And Inequalities Quiz Part 2
The steps for solving absolute value equations and inequalities are similar. However, there are some key differences. The table below compares the steps for solving absolute value equations and inequalities.
| Step | Absolute Value Equation | Absolute Value Inequality |
|---|---|---|
| 1 | Isolate the absolute value expression on one side of the equation. | Isolate the absolute value expression on one side of the inequality. |
| 2 | Use the properties of absolute value to solve for x. | Use the properties of absolute value to solve for x. |
| 3 | Check your solution by plugging it back into the original equation or inequality. | Check your solution by plugging it back into the original equation or inequality. |
Here are some examples of absolute value equations and inequalities:
- Solve the equation |x| = 5.
- Solve the inequality |x| < 3.
- Solve the equation |2x – 5| = 7.
- Solve the inequality |3x + 1| > 4.
Applications of Absolute Value Equations and Inequalities
Absolute value equations and inequalities have many applications in real-world situations. For example, absolute value equations can be used to find the distance between two points on a number line. Absolute value inequalities can be used to find the range of values that a variable can take on.
Here are some examples of real-world applications of absolute value equations and inequalities:
- A company wants to build a new warehouse that is no more than 5 miles away from its headquarters. The company is considering two locations for the warehouse. Location A is 3 miles away from the headquarters, and Location B is 7 miles away from the headquarters.
Can the company build the warehouse at Location B?
- A store is selling a shirt for $20. The store is offering a discount of $5 on the shirt. What is the range of prices that the shirt can be sold for?
Question & Answer Hub
What is an absolute value equation?
An absolute value equation is an equation that contains an absolute value expression. The absolute value of a number is its distance from zero on the number line. For example, the absolute value of 5 is 5, and the absolute value of -5 is also 5.
What is an absolute value inequality?
An absolute value inequality is an inequality that contains an absolute value expression. For example, the inequality |x| > 5 means that the distance between x and 0 is greater than 5.
How do you solve absolute value equations?
To solve an absolute value equation, you need to isolate the absolute value expression. Then, you can use the properties of absolute value to solve the equation. For example, the equation |x| = 5 can be solved by setting x = 5 or x = -5.
How do you solve absolute value inequalities?
To solve an absolute value inequality, you can use the properties of absolute value to rewrite the inequality in a more manageable form. Then, you can use the properties of inequalities to solve the inequality. For example, the inequality |x| > 5 can be rewritten as x > 5 or x< -5.
